<-CHM151 Progress page
Chapter 7 Textbook Readings and Problems
ATOMIC STRUCTURE
Last update (11/20/09)

CHAPTER 7 TEXTBOOK READINGS
ENGINE

For all editions

You are welcome to read all sections in this chapter, but I think the tutorials I did on the first sections of this chapter are adequate.

Read the sections labeled:
Periodic Trends in Atomic Properties.

The Properties of a Group: The Alkali Metals
PROBLEMS
The problems were taken from the 6th, 7th, and 8th editions. You don't have to find them in your textbook because I've reproduced them below.
balloon

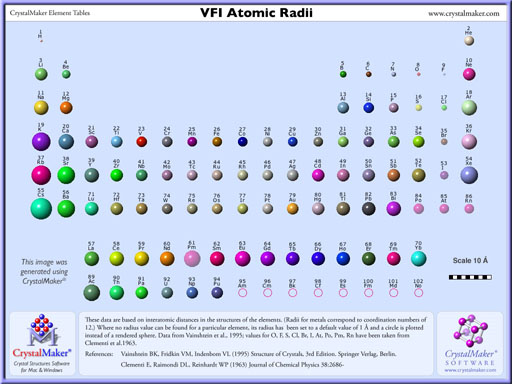
Problem 1: Arrange the following groups of atoms in order of increasing size.
a. Te, S, Se
b. K, Br, Ni
c. Ba, Si, F

The textbook has an image that shows the sizes. The below link has a chart that shows the radii of all elements. Note that the radius is written above the element. Or click on the image to the left to see a large version of that table (same as on the web page link below).
http://www.standnes.no/.../atomic-radius-elements.htm
Elements that have the small ionization energy are those who give up their electron easily. The alkali metals and the alkaline earth metals are ones known for loosing their electrons easily and becoming positive.
Problem 2: In each fo the following sets, which atom or ion has the smallest ionization energy (first electron).
a. Ca, Sr, Ba
b. K, Mn, Ga
c. N, O, F
d. S2-, S, S2+
e. Cs, Ge, Ar
The textbook should have this info. Also, you can visit www.webelements.com link below and find a chart like one shown where you can roll cursor over the element and read the ionization values. It won't have ions like in (d), but you can figure that out without looking it up.
http://www.webelements.com/periodicity/ionisation_energy_1/

wave function

The textbook listed the complex equation to the left saying it was the wave function for hydrogen for the p orbital for n=2 (2nd shell). The z in 2pz is for the p orbital that aligns with the z axis (up and down double-lobe in image). They want you to solve the equation and then square it. Squaring these wave functions gives you the probability of the electron being at that location. The big "Z" is the atomic number. Since this is for hydrogen, it is "1". They say the σ (sigma) symbols equals Z(r/a0) where a0 is the radius of the Bohr orbit (the normal distance electron is from the nucleus). That value is given as 5.29x10-11 meters. They want you to solve the equation when r (current radius of electron) equals to a0 (the normal radius). "e" is called the natural logarithm. The value is approximately 2.718. Also, they said to use the angle of 0° and of 90°. Zero degrees means it is on the z axis. 90° means it is on the xy plane. In the image, the p orbital on the z axis is yellow. When the angle is 90°, it lies on the xy plane. You should see that the 2 yellow spherical areas do not touch the xy plane. So that answer will be zero. Also, cosine of 90° is zero, which makes the whole calculation zero. I don't think I would want to solve this equation using a calculator. Even with a spreadsheet it's rather difficult, but lets try it below.
wave function
 
A
B
C
D
E
F
G
H
I
 
Z is atomic # (hydrogen=1)
              
1
Z=
 1
(Bohr's radius) a0=
=5.29*10^-11

meters

 This is where the electron spends most of its time
2
σ=Z(r/a0)=
 =B1*(D2/D1)
(current radius) r=a0=
=5.29*10^-11
angle θ=
0
 deg    
3
If r=a0, then r/a0=1. Also Z=1, so σ=1 under these conditions.
  
Note:
cos 0°= 1
    
Probability of electron at this location
4              
5  
1/4√2π
(Z/a0)3/2
σ
e-σ/2
cos θ
  
Ψ2pz
(Ψ2pz)2
6
Ψ2pz=
1
=(B1/D1)^(3/2)
=B2
=exp(1)^(-B2/2)

=cos(F2)
=
 =C6*D6*E6*F6/B7
=H6^2
7

=4*sqrt(2*pi())

              

Problem 3: How do you enter π (pi) into a spreadsheet?
Problem 4: You can get a square root in a spreadsheet by setting exponent to 0.5. There's also a square root function. How is that written in a spreadsheet?
Problem 5: The textbook said to also solve the equation when the angle is 90°. You would think to just enter 90 in the angle cell above (F2), however, be aware that most spreadsheets and most calculators, don't use degrees. You are familiar with a circle being 360°, but in math it is more common to measure that circle by the distance around a circle that has a radius of 1. That distance is 2 x π. 90° is a quarter of a circle, so we have to take 1/4 of 2π, which equals π/2. So that's the angle put into F2 instead of 90°. From what learned from Problem 3, how do you enter π/2?

a
 b
c
d
(Continuation of above problem). On the left are representations of the p orbital. The left is the region where the electron spends 90% of its time. The right half has points spread out according the probability of where the electron is. The red dot is about where the electrons spends most of its time. That's known as the Bohr radius. The answer to psi (Ψ) squared above was 2.47x1028, which represents the probability of the electron being that that spot. That doesn't mean much unless you have something to compare it to. So I created 20 rows of the above calculations and solved for the radius being about 50 times farther out from red dot down to near the nucleus. The values peaked at the Bohr radius of 5.29x10^-11 meters. As you change the radius (r) in the formula to go farther out, the probability drops off quickly. It looks like the curve at the top of the blue sphere. As the radius (distance from center) got smaller it slowly dropped and then dropped very quickly. Again, it matched the curve at the bottom of the blue sphere. The bottom orange sphere is just the other half of the p orbital.
Problem 6: This p orbital is 2p (having quantum numbers of n=2, l=1, m=0. Visit this website and read the overview. http://www.orbitals.com/orb/ Then find the link to the "Grand Table" and check out the grand table of orbital images. Find the orbital image for n=3, l=1, m=0. Which image on the left matches what you find?
Send your answers to CHM151@chemistryland.com. Use Subject title of "Chapter 7".

<-CHM151 Progress page

Since Nov 17, 2009